Shape Transformer
Primary (Age 6 – 9)
Curriculum Goal
Primary: Geometry and Spatial Sense
- Give and follow multistep instructions involving movement from one location to another, including, distance, half- and quarter-turns.
Context
- Students should understand directional and rotational language. Some knowledge of angles (90 degrees, 180 degrees, etc.) would be helpful.
Materials
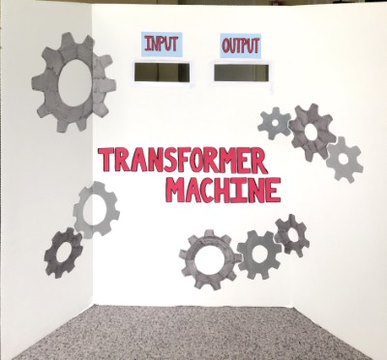
- Transformer Machine display board (with cutouts for “input” and “output” slots – see Figure 1)
- Introductory slides (Appendix A)
- Paired input/output cards (Appendix B)
- A place to display the paired cards in two columns (on white board, the carpet, or chart paper – see Figure 2)
Introduction
- Introduce students to the Transformer Machine (see Figure 1) and explain that the machine operates with an ‘input’ and produces an ‘output’ that has gone through a specific transformation. For example, an input shape will be inserted, and the output of that shape is the same shape, but 90 degrees rotated clockwise (see Figure 2).
- Students take on the role of “detectives” to solve and accurately describe the Transformer Machine’s transformation rule.
- Note to educator: This lesson can be conducted using the slide deck to project for students in-person, or for online learning. Alternatively, your own Transformer Machine can be created to use in the classroom with students.
Lesson
Using the Slide Deck (Appendix A):
- Using the introductory slides (Appendix A), show students the concept of the Transformation Machine: there is an input that goes through a transformation to produce an output. Explain to students that a transformation can include any change made to the input card.
- Have students brainstorm a list of potential changes that might be made to an input. Students might say that the input shape could be rotated, flipped, a mirror reflection, etc.
- Go through the first five examples on the slides with the students. Have them observe the input image with its corresponding output image and guess the transformation rule.
- After hearing several guesses, write the correct and specific rule for each of the examples included for students to confirm their guesses.
- Go through the next two examples on the slides, taking the time to explain the table that displays the paired inputs and outputs. Ask students to observe the table and share what they notice about the inputs and outputs.
- Students should notice that all the inputs and outputs follow the same transformation rule.
- Have several students guess the rule and then share the correct transformation rule.
- Have students check their understanding of the last two examples in the slide deck. When having students predict the outputs of shapes based on the transformation rule, ask them to first visualize in their mind’s eye – encourage students to close their eyes and mentally take the input and transform it according to the observed rule – and then share the output.
Using the physical Transformer Machine:
- Introduce students to the physical Transformer Machine. Present students with an input card and insert it into the Transformer Machine through the input slot (Figure 2). Take the corresponding output card and withdraw it through the Machine’s output slot (Figure 3).

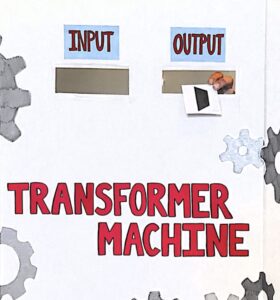
- Place the pair of cards next to each other in two columns called “Input” and “Output” for the students to see (Figure 4).
- If possible, present at least three example pairs of inputs and outputs that follow the same rule. This allows students to observe the pattern and arrive at a transformation rule that generalizes to the multiple examples shown.
- Have several students share their guess of the transformation rule using verbal description, and then describe the specific transformation rule modeling precise rotational and spatial language.
- After a few examples of this, have students visualize in their mind’s eye and draw the output of a given input card before its corresponding output card is revealed.
Conclusion
- Have students create a table of paired inputs and outputs based on coming up with their own transformation rule.

Look Fors
- Are students recognizing the specific transformations occurring to the output shapes compared to the corresponding inputs?
- Are students using positional language correctly? Are they building on one another’s ideas using additional spatial language to describe the transformations?
- Are students visualizing the change occurring to the input card based on the specific transformation rule?
Extension
- Use numbers as inputs and outputs instead of shapes.
Share this lesson
Share on facebook
Share on twitter
Share on email