Visual Multiplication

Junior (Age 9 – 12)

Curriculum Goal

Junior: Number Sense

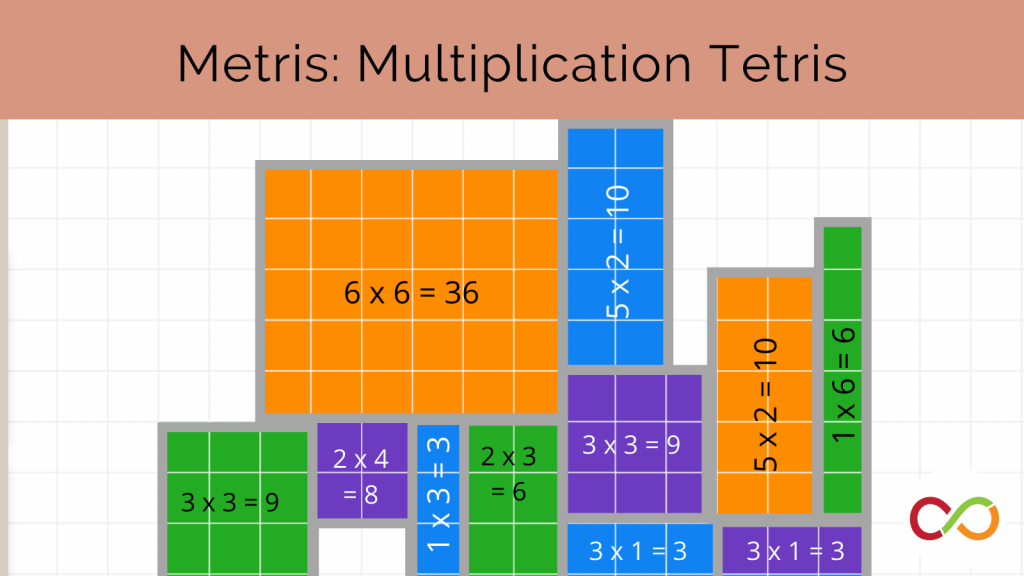
Represent and solve problems involving the multiplication of two-digit whole numbers by two-digit whole numbers using the area model and using algorithms, and make connections between the two methods.

Context

Teacher-led, whole-class introduction followed by an exploratory pair-based activity that strengthens students’ understanding of multiplication.

Materials

Instructions for visual multiplication (Appendix A)
Large chart paper
Markers
Paper and pencil crayons for each student
Multi-coloured sticks/straws (or something similar)

Lesson

Give students one minute to draw or write down anything they know about multiplication on large chart paper.
- Suggested questions:
  - What is multiplication?
  - What do you know about multiplication?
  - How do you solve multiplication problems?
  - Do you use any “tricks” to solve multiplication problems?
Have students share ideas with the class and highlight central ideas in multiplication, such as arrays and repeated addition.
Background information on this multiplication strategy is available at the end of this lesson plan.
Introduce visual multiplication using coloured lines to show place value (Appendix A).
Ensure you explain each step with visuals to the students. Demonstrate how you would do a one-digit by one-digit question, as well as a two-digit by two-digit question.
- Explain that the sticks represent the digits of each number and that the intersections of the sticks are counted in a particular way to get your product. Please refer to examples one and two in the resource from The University of Georgia for additional guidance (see More Information below).
Introduce three-digit by three-digit questions when your class is ready.
Challenge students to answer a few questions together as a group. Begin with one-digit by one-digit questions then move to two-digit by two-digit questions. Once proficient, have them pair up and challenge each other.
When students have had an opportunity to practice this method, discuss as a group whether they would use this method of multiplication.
- Challenge students to think about when this method is helpful and when it might not be (e.g., When is this method more laborious than algorithms you know?).
- Ask students to explain how this method works.
- Ask students whether there is a limit to this way of multiplication?

Look Fors

Are students able to draw out the multiplication questions using the visual multiplication method?
Are they able to group the intersections together? Do the students understand what the intersection of sticks represents?

Extension

Visual Multiplication Further Explained
In this video from tecmath, they break down each step required to multiply two-digits by two-digits using this method. They also breakdown how to multiply three-digit by two-digit numbers.

“Chinese Stick Multiplication”: Additional resource
This article from the Mathematics Education program at the University of Georgia breaks down the steps when multiplying one-digit by one-digit numbers, two-digit by two-digit numbers and then three-digit by three digit-numbers using this method. The author has included visual examples to show you exactly how to group the intersections of sticks to find your products.

Related Lessons

Students work collaboratively to understand multiplication by using the concepts of length, width, and area of shapes.

Possible introductory activity: Students engage in a game to recall and demonstrate one-digit multiplication facts.